A new math
Moderator: Moderators
A new math
Post #1This topic is for discussing errors in our current understanding of math.
"I believe in no religion. There is absolutely no proof for any of them, and from a philosophical standpoint Christianity is not even the best. All religions, that is, all mythologies to give them their proper name, are merely man’s own invention..."
C.S. Lewis
C.S. Lewis
- Divine Insight
- Savant
- Posts: 18070
- Joined: Thu Jun 28, 2012 10:59 pm
- Location: Here & Now
- Been thanked: 19 times
Post #31
I'm not straw manning you at all John. You're just not understanding what I'm saying and why I began with my conditional statement.JohnA wrote: The bit that you refuse to accept is that you are straw manning me. I do not have to accept or reject your P. That is because you fail at the logic bit. Trying to use math to show math is fallacious, it's a fundamental failure of logic on your part. I can explain it to you, but I can not make you understand it. That is your job.
My conditional statement has nothing at all to do with any proofs, arguments, or logic involved in demonstrating any conclusions about mathematics. The mere fact that you think it does only reveals that you don't understand what I'm saying at all.
All I'm saying in this conditional statement is that I have no desire to waste time arguing with anyone who doesn't already accept that mathematics should correctly describe the quantitative nature the physical universe.
That's all this conditional statement is saying. Period.
Why would I want to bother arguing with people who aren't at least on the same page that I'm on?
I offer this conditional statement for one reason and one reason only: To weed out people who are going to argue that mathematics doesn't need to correctly describe the quantitative nature of physical reality.
In other words, if you reject the hypothesis of my conditional statement then you are on an entirely different page from me. You clearly aren't concerned with whether mathematics correctly describes physical reality or not.
So why would I even want to bother discussing this topic with you?
You have already shown that you aren't even interested in accepting my prime hypothesis.
That is the ONLY PURPOSE of this conditional statement John. It's doesn't "prove" anything about anything. It's not a logical conclusion. It can either be true or false at this point.
If you reject the hypothesis then look at the Truth Table John. You have accepted the truth of the conditional statement by default.
The only way you can argue that the statement is false it to assert that P is TRUE and Q is FALSE. And it is only those people that I'm interested in debating with.
You have already rejected the truth of P, therefore you're out. You're in a group that I don't care to discuss the matter with. Apparently you are happy with a mathematics that does not correctly describe the true nature of the physical universe.
And that's fine. There's no problem with that. I just have no reason to argue with you on that. If you're happy with P being false, then more power to you. I'm not going to waste my time trying to convince you otherwise, because at that point we'd just be arguing subjective philosophical opinions. Something that even you claim to reject as "rubbish".
I don't want to waste my time on that "rubbish" either. And that's precisely why I have constructed my conditional statement exactly as I have constructed it. I did this specifically to weed out useless philosophical arguments that amount to nothing more than subjective opinions.
You can't even get past this initial simple thing. I'm not sure if I would even want to bother discussing the rest of it with you even if were you to accept the truth of P at this point. You have already demonstrated to me that you have trouble understanding the simplest ideas.
My conditional statement is simply stating that I'm not interested in discussing the matter with anyone who rejects P.
That's all it's saying and it's not making any statements or conclusions about mathematics in general at all. Before it can do that P must be accepted as being TRUE and then a demonstration that Q is either True or False must follow that.
But you have rejected P already, so you're done.
Look at the truth table John:
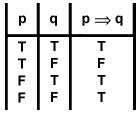
Once you have rejected the truth of P, the truth value of Q no longer matters.
[center]
Spiritual Growth - A person's continual assessment
of how well they believe they are doing
relative to what they believe a personal God expects of them.
[/center]

Spiritual Growth - A person's continual assessment
of how well they believe they are doing
relative to what they believe a personal God expects of them.
[/center]
- Divine Insight
- Savant
- Posts: 18070
- Joined: Thu Jun 28, 2012 10:59 pm
- Location: Here & Now
- Been thanked: 19 times
Post #32
That's certainly a far better start.micatala wrote: OK, let's just consider DI's conditional statement. I think P is false, but I will set that aside for the time being.
However, the mere fact that you are actually happy with P being false suggests to me that you will still be "hostile" to my position. But at least you're recognizing the importance of accepting the truth of this hypothesis.
Yes it's "logically inconsistent" but only within the framework of accepting the truth of P. After all, if you reject P then the logical inconsistencies vanish.micatala wrote:I need a precise definition of "wrong.""IF modern mathematical formalism is supposed to correctly describe the quantitative nature of our physical universe, THEN our current modern mathematical formalism is wrong".
Do you mean "logically inconsistent?"
Why should that be? Well, if mathematics is supposed to correctly describe the quantitative nature of the physical universe, then if I can show that it deviates from this, that would then be a "logical inconsistency" in this context. And that is precisely where I'm headed.
On the other hand if you don't demand that mathematics must correctly describe the quantitative nature of the physical universe, you can argue that the logical inconsistencies I provide are not relevant.
This is why we must always keep the truth of P foremost in everything we consider. As soon as you dismiss the truth of P, then you back off in another ball park entirely.
I do believe that I can demonstrate this as well. However, this may require some preliminary groundwork.micatala wrote: Or, do you by contrast mean that mathematics makes assertions that are contrary to other known facts, either about the physical world or not?
Nope, you've pretty much hit the nail on the head.micatala wrote: Do you mean something else altogether?
I should be able to, but unfortunately to do this would conflict with much of currently accepted mathematics, which people then instantly become defensive about.micatala wrote: You should be able to provide a fairly simple, short paragraph definition of what exactly you mean, here.
But I'll try to summarize what went wrong historically in bullet form. How's that?
To begin with mankind started to explore the quantitative nature of the universe by counting things. This was originally done to simply keep track of commodities, or simply days, months, or years.
For example, pebbles may have been used to keep track of how many sheep a Shepard owns. Each individual pebble was matched up with each individual sheep and placed into a bag. When the sheep were brought in for the night the Shepard could match up the pebbles with the sheep to see if all the sheep were home safe.
This was a one-to-one and onto correspondence of physical individual objects.
Now here's a question for you: "Was mathematics abstract at this point?"
My answer to this question is, "Yes it was". It was already an abstract formalism because you could use pebbles to keep track of sheep, or goats, or how many fish you caught, or how many seed you planted, or crops you harvested, etc.
It was already abstract at that point. Yet both the pebbles and the things being matched up with the pebbles were physical objects that have well-defined properties of individuality. And this was the basis of the concept of "number".
There was no need to abstract this concept further.
But we did abstract it further. One place we might think to look for further abstracting was the keeping of time. In the very early days time was typically kept by marking notches on a stick. These notches would record each sun rise, or may be each sun set. Or maybe each full moon to keep track of moonths.
Or maybe the notches were placed on a very special stick that kept tract of seasons, often that was done once a year at the winter Solstice to keep track of the yearly cycle of the Sun.
Now that question becomes, "Did these intervals of time have a well-defined physical property of individuality?"
My answer to this question is, "Yes they did." Their well-defined property of individuality come from the physical fact that they are indeed very consistent periodic motions that can be recognized at very specific places in their periods.
So, this was not additional "abstraction". It's still precisely the same kind of abstraction applied to stones, sheep, goats, fish, etc. Everything at this point is physically defined. It's all a physical property of physical reality.
But now we're about to move into an area where the physical properties of physical reality are not so readily apparent.
Men started to draw figures and measure distances in arbitrary units. It was believed that these arbitrary "units" could indeed be made physical. Just build a ruler and there you have it, and arbitrary "unit" of distance.
So they started to get into geometry and measuring properties of squares, circles, and other geometric figures. Soon they realized that some of the properties of these geometric figures could not be commensurate with a single unit of distance measure. This totally blew their minds and the didn't know how to do with it. So they invented the concept of an irrational quantity.
Now to this very day people will argue that irrational quantities necessarily must exist, because things like circles, squares, and other such situation actually exist in the real world.
But is that a valid conclusion?
I say no, it is not a valid conclusion at all.
And we especially know that it's not valid today in light of Quantum Mechanics. Even the physical universe herself "rounds off" at the quantum level. To demand that the diagonal of a physical square, or the diameter of a circle must be carried out to infinite precision thus producing an irrational quantity, is simply not part of how our universe physically behaves. Our physical universe "rounds off" at the quantum level. Somewhere at the Planck Length.
These "irrational quantities" are not an absolute quantitative property of our universe. Instead what they are, are the result of self-referenced, or self-dependent situations. Moreover, not only is this the truth of the physical universe, but we can even learn something about the universe by recognizing this physical behavior.
~~~~~~~
Ok, that's more than a short paragraph, and I didn't even cover why a set theory based on an empty set totally ignores these facts and instead describes something totally different from physical reality.
But there you go. We simply got off track mainly because we didn't understand how to handle irrational relationship. We are trying to handle them as the cardinal property of a collection of individual things and that leads to absurdities that have absolutely nothing at all to do with the quantitative nature of the physical universe in which we live.
Is a set theory based on an empty set "logically consistent"? Actually I would personally say that it isn't and I could give arguments for that, but I'm really not interested in going there. But even if it were "logically consistent", that still doesn't mean that it would be a correct description of the quantitative nature of our physical universe.
It would seem to me that any physical scientists would be more interested in a mathematics that actually correctly describes the physical world in which we live.
A mathematics that is also a science.
[center]
Spiritual Growth - A person's continual assessment
of how well they believe they are doing
relative to what they believe a personal God expects of them.
[/center]

Spiritual Growth - A person's continual assessment
of how well they believe they are doing
relative to what they believe a personal God expects of them.
[/center]


